Exemplary Info About What Does A Smooth Curve Show How To Set Up Line Graph In Excel

A smooth curve is a curve which is a smooth function, where the word curve is interpreted in the analytic geometry context.

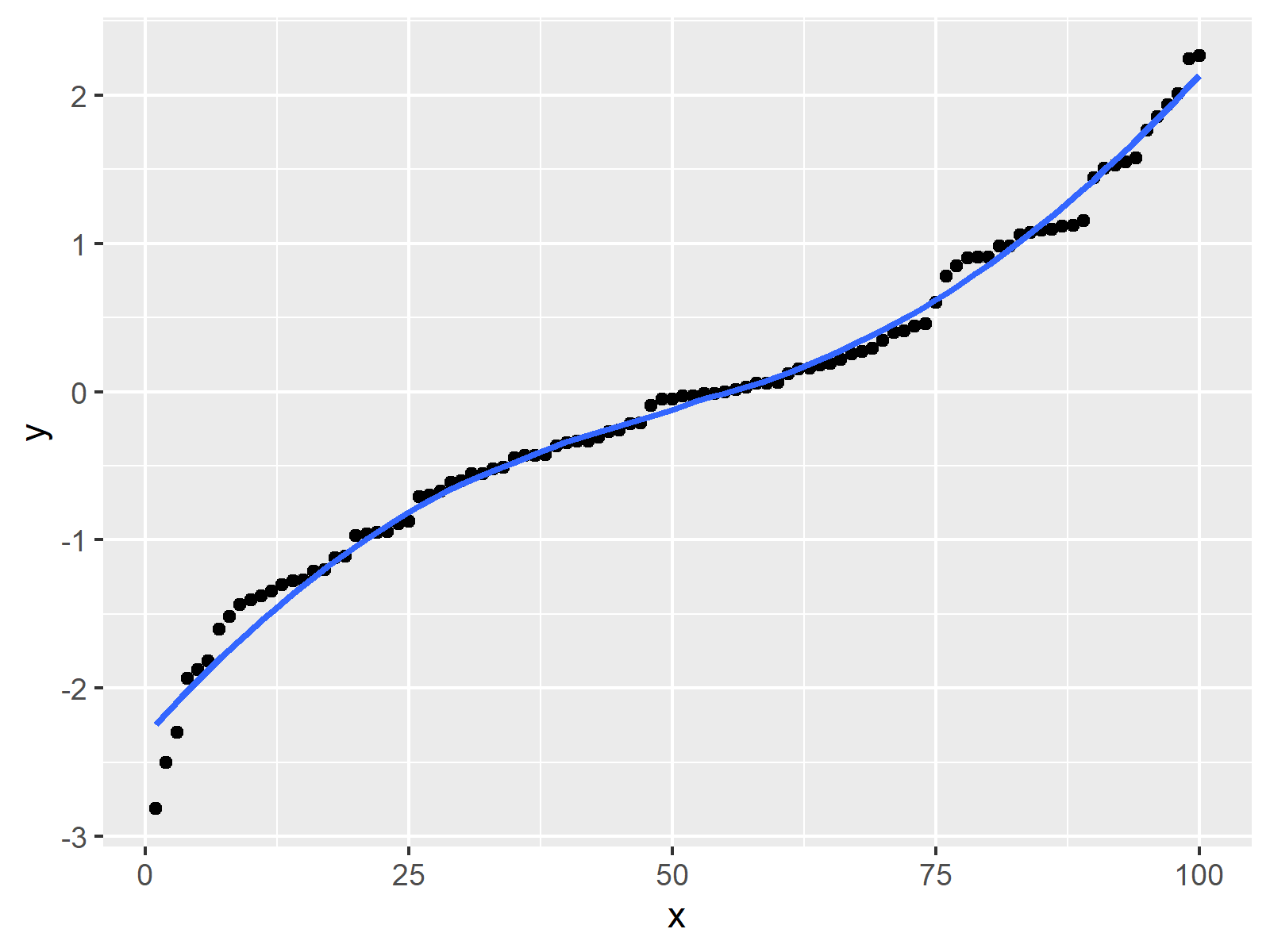
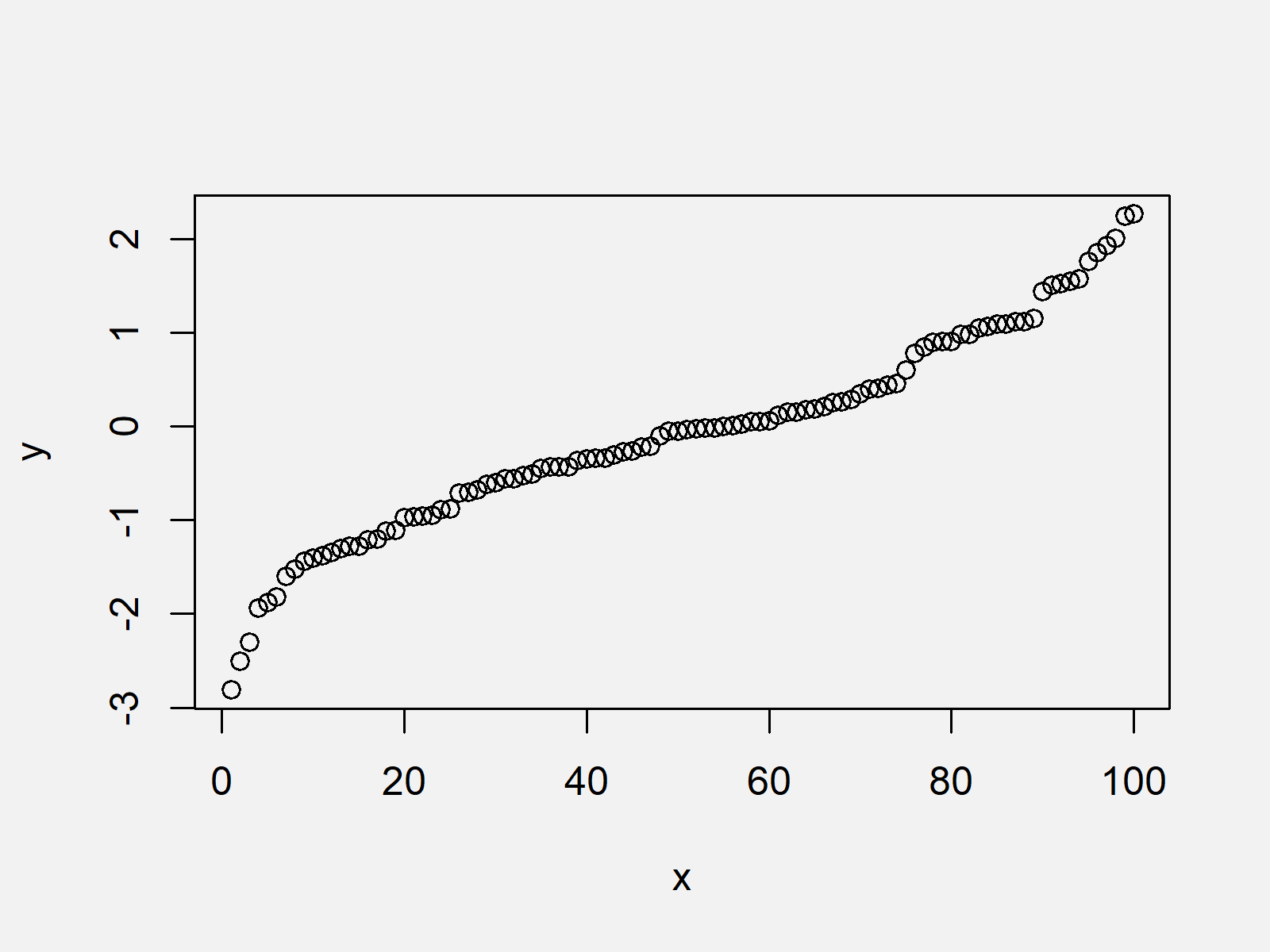
What does a smooth curve show. Modified 9 years, 1 month ago. A field will be established in the wire, and electrons. In the early days of design, a smooth curve was manually drawn through a given set of points by placing metal weights, called “ducks,” at the data points, and then passing a.

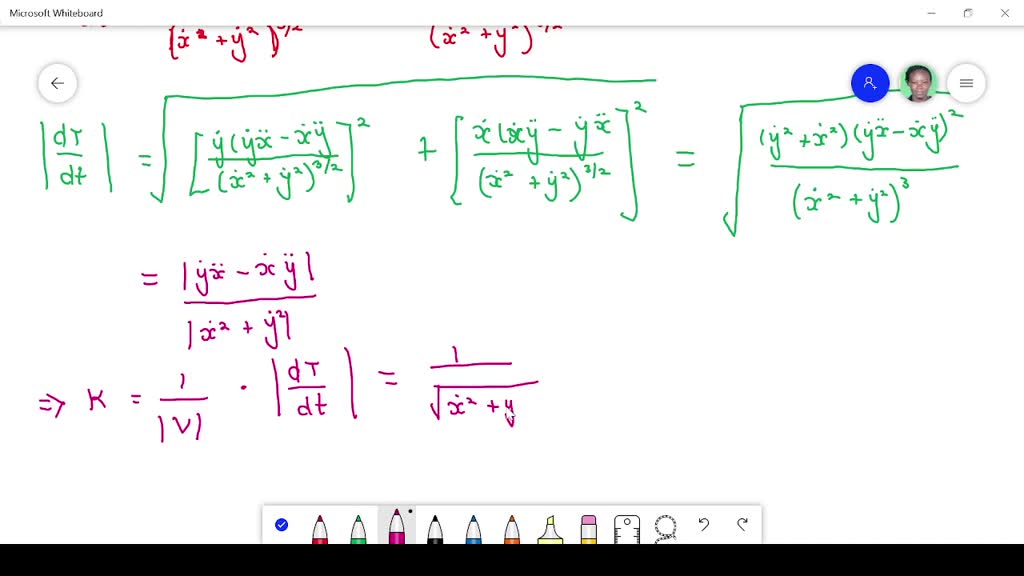
Whats the definition for $r$ to be smooth? In this section we want to briefly discuss the curvature of a smooth curve (recall that for a smooth curve we require →r ′(t) r → ′ ( t) is continuous and →r ′(t) ≠ 0 r. A smooth curve can be expressed as a smooth projective curve of genus g minus r points, with g and r unique?

This looks to me very. To showcase the behaviour of the different methods in the middle of the data. A curve $\gamma\colon i \to \mathbb{r}^n$ is an immersion iff it is $c^\infty$.

The different savgol and average filters produce a rough line, lowess, fft and kernel. Yy(1) = y(1) yy(2) = (y(1) +. The curve α(t) = (t3, t2) in the plane fails to be.

Complex) solution than (0, 0, 0). Lowess (locally weighted scatterplot smoothing), sometimes called loess (locally weighted smoothing), is a popular tool used in regression analysis that creates a smooth. In particular, a smooth curve is a.

A smooth curve is any curve for which r ˙(t) r → ˙ ( t) is continuous and r ˙(t) ≠ 0 r → ˙ ( t) ≠ 0 for any t t except possibly at the endpoints. In this video, i show that a curve described by a vector function is not smooth by showing there are values of t that make the derivative equal to zero. Suppose γ γ is simple smooth.

A curve $\gamma\colon i \to \mathbb{r}^n$ is smooth iff it is $c^\infty$ (or $c^p$ for some authors). Let $r :\mathbb{r}^1\rightarrow \mathbb{r}^n$ be a representation of a curve. If you import a curve from an instrument, you may wish to smooth the data to improve the appearance of a graph.

Complex) projective plane if the system has no other real (resp. Equivalently, we say that α is an immersion of i into r3. Consider the following curve in the plane, $(x(t),y(t))$, this curve is called smooth if the functions $x(t)$ and $y(t)$ are smooth, which simply means that for all $n$, the derivatives $\frac{d^nx}{dt^n}$ and $\frac{d^ny}{dt^n}$ exist.

Yy = smooth(y) smooths the response data in column vector y using a moving average filter. Asked 9 years, 1 month ago. Basic properties of smooth curves.

The first few elements of yy follow. A smoothness property for a function with values in rn r n, by definition, is equivalent to that same smoothness property holding. A smooth curve α: